IMS2024 Conference Themes

Title

Solution to Last Month’s Quiz

Takashi Ohira

Figure 1 shows the circuit diagram for last month’s enigma. The rectifier is excited by a purely sinusoidal voltage source vs(t). However, the diode nonlinearity makes the current is(t) contain the dc component Io plus multiple harmonics [denoted by ${\cdots}$ in (2)] in addition to the fundamental wave as follows: \begin{align*}{v}_{s}{\left({t}\right)} & = {\left[\begin{array}{cc}{V}_{P}&{V}_{Q}\end{array}\right]}{\left[\begin{array}{c}{\sin}\,{\omega}{t} \\ {\cos}\,{\omega}{t}\end{array}\right]} \tag{1} \\ {i}_{s}{\left({t}\right)} & = {I}_{o} + {\left[\begin{array}{cc}{I}_{P}&{I}_{Q}\end{array}\right]}{\left[\begin{array}{c}{\sin}\,{\omega}{t} \\ {\cos}\,{\omega}{t}\end{array}\right]} + {\cdots}{.} \tag{2} \end{align*}

Figure 1. The rectifier circuit diagram.

Focusing on the fundamental wave term in (2), the trigonometric Fourier theorem tells us \[{\left[\begin{array}{c}{I}_{P} \\ {I}_{Q} \end{array}\right]} = \frac{2}{T} \mathop{\int}\nolimits_{0}\nolimits^{T / 2}{i}_{s}{\left({t}\right)}{\left[\begin{array}{c}{\sin}\,{\omega}{t} \\ {\cos}\,{\omega}{t}\end{array} \right]}{dt}{.} \tag{3} \]

Since we assume half-wave rectification (50% duty cycle), this integral is not for the entire period T but confined to the half interval 0 < t < T/2 only when the diode stays on.

The previous puzzles [1], [2] remind us that \[{i}_{s}{\left({t}\right)} = \frac{\left[{V}_{P} \quad {V}_{Q}\right]}{{\omega}{L}}{\left[\begin{array}{c}{1} - {\cos}\,{\omega}{t} \\ {\sin}\,{\omega}{t}{-}{\omega}{t}\end{array}\right]} \tag{4} \] \[{V}_{P} = \frac{\pi}{2}{V}_{Q} \tag{5} \] \[{V}_{Q} = {V}_{o} = {R}_{o}{I}_{o}{.} \tag{6} \]

Adopting (4)–(6) into (3), the half-wave integral yields \begin{align*}{\left[\begin{array}{c}{I}_{P} \\ {I}_{Q} \end{array}\right]} & = \frac{1}{2{R}_{o}}{\left[\begin{array}{cc}{4}&{{-}{\pi}}\\{{-}{\pi}}&{4}\end{array}\right]}{\left[\begin{array}{c}{V}_{P}\\{V}_{Q}\end{array}\right]} \\ & = \frac{1}{4}{\left[\begin{array}{c}{2}{\pi} \\ {8}{-}{\pi}^{2}\end{array}\right]}{I}_{o}{.} \tag{7} \end{align*}

The first row of (7) finds IP = (π/2)Io. Therefore, the correct answer to last month’s quiz is (c).

Equations (5), (6), and (7) merge into a single matrix form: \[{\left[\begin{array}{cc}{V_P} & {I_P} \\ {V_Q} & {I_Q} \end{array} \right]} = \frac{1}{4} {\left[\begin{array}{cc}{2}{\pi}{R_o} & {2}{\pi} \\ {4}{R_o} & {8} - {\pi}^{2} \end{array} \right]}{I_o}. \tag{8} \]

As seen in (8), all of the RF input components have been solved back from the dc output current. Thus, we are ready to reveal the rectifier’s practically important properties, namely, input impedance and power conversion efficiency. They will be coming up step by step in forthcoming enigmas.

References

[1] T. Ohira, “Rectifier output voltage,” IEEE Microw. Mag., vol. 24, no. 3, p. 99, Mar. 2023, doi: 10.1109/MMM.2022.3226632.

[2] T. Ohira, “Half-wave rectification,” IEEE Microw. Mag., vol. 24, no. 5, p. 149, May 2023, doi: 10.1109/MMM.2023.3242520.

Digital Object Identifier 10.1109/MMM.2023.3294882

IEEE microwave magazine   October 2023

Title


Editor’s Note: This conference information is accurate as of the content deadline for the IEEE Microwave Magazine.

OCTOBER 2023

2023 IEEE Global Humanitarian Technology Conference (GHTC)

12–15 October 2023

Location: Radnor, PA, USA


2023 IEEE BiCMOS and Compound Semiconductor Integrated Circuits and Technology Symposium (BCICTS)

14–18 October 2023

Location: Monterey, CA, USA


2023 International Topical Meeting on Microwave Photonics (MWP)

15–18 October 2023

Location: Nanjing, China


2023 IEEE 32nd Conference on ­Electrical Performance on Electronic Packaging and Systems (EPEPS)

15–18 October 2023

Location: Milpitas, CA, USA


15th International Conference on Advanced Technologies, Systems, and Services in Telecommunications (TELSIKS)

25–27 October 2023

Location: Nis, Serbia

NOVEMBER 2023

2023 SBMO/IEEE MTT-S International Microwave and Optoelectronics ­Conference (IMOC)

5–9 November 2023

Location: Castelldefels, Spain


IEEE Conference on Microwaves, Communications, Antennas, Biomedical Engineering, and Electronic Systems (IEEE COMCAS 2023)

6–8 November 2023

Location: Tel Aviv, Israel


2023 International Workshop on ­Integrated Nonlinear Microwave and Millimeter-Wave Circuits (INMMIC)

8–11 November 2023

Location: Aveiro, Portugal


2023 IEEE MTT-S International Micro­wave Workshop Series on ­Advanced Materials and Processes for RF and THz Applications (IMWS-AMP)

13–15 November 2023

Location: Chengdu, China

DECEMBER 2023

2023 Asia-Pacific Microwave Conference (APMC)

5–8 December 2023

Location: Taipei, Taiwan


2023 IEEE MTT-S Latin American Microwave Conference (LAMC)

6–8 December 2023

Location: San Jose, Costa Rica


2023 IEEE Microwaves, Antennas, and Propagation Conference (MAPCON)

11–14 December 2023

Location: Ahmedabad, India

JANUARY 2024

2024 IEEE Radio and Wireless Week (RWW) (PAWR, RWS, SiRF, SHaRC, WiSNet, colocated with ARFTG)

21–24 January 2024

Location: San Antonio, TX, USA

February 2024

2024 International Microwave Filter Workshop (IMFW2024)

21–24 February 2024

Location: Cocoa Beach, FL, USA

APRIL 2024

2024 IEEE Wireless and Microwave Technology Conference

15–16 April 2024

Location: Clearwater Beach, FL, USA


2024 IEEE International Conference on Microwave for Intelligent Mobility (ICMIM)

16–17 April 2024

Location: Boppard, Germany

June 2024

International Microwave Symposium (IMS, colocated with RFIC, ARFTG)

16–21 June 2024

Location: Washington, DC, USA

IEEE microwave magazine   October 2023

Title

State of the Industry: Diversity in the Workplace

Sherry Hess

I recently wrote a Women in Microwaves (WIM) column for this magazine based upon my prior involvement at the Women in Engineering panel discussion held at the 2022 Design Automation Conference (DAC) last summer. That article was titled “Real Advice for Today’s Women in Engineering,” and the discussion centered around the history and experience of the panelists Geeta Pyne of Intuit, Susanna Holt of Autodesk, Radhika Shankar of Synopsys, and myself (Sherry Hess of Cadence), as women in the male-dominated world of engineering.

Moderator Ann Steffora Mutscher of Semiconductor Engineering opened with comments gleaned from two recent articles on diversity in the workplace and why it is so important. The NeuroLeadership Institute article states in “Why Diverse Teams Outperform Homogeneous Teams” [1] that studies show that diverse teams think more logically, are more creative, and are more adept at identifying errors in thinking. “We know that when the stakes are high—we’re relying on the development of an innovative product or idea, we’re grappling with uncertainty, or a crisis is bearing down—diverse teams are critical.”

The Harvard Business Review article “Why Diverse Teams Are Smarter” [2] says, “In a nutshell, enriching your employee pool with representatives of different genders, races, and nationalities is key for boosting your company’s joint intellectual potential. Creating a more diverse workplace will help to keep your team members’ biases in check and make them question their assumptions. At the same time, we need to make sure the organization has inclusive practices so that everyone feels they can be heard. All of this can make your teams smarter and, ultimately, make your organization more successful, whatever your goals.”

Despite More Diversity Awareness, Women Are Leaving

In light of the information in these articles, which reflects the same research I have shared in various articles and blogs over the years, it was disheartening to discover a report published last fall by McKinsey and Co. for Women in the Workplace in partnership with Lean In (https://leanin.org/) that says the needs of women in the workplace are by and large not being met, and they are leaving in unprecedented numbers, both older, experienced women in leadership roles and younger women just embarking on their careers. Because women are already significantly underrepresented in leadership roles, this “flight” only puts us further behind in efforts to provide diversity, equality, and inclusion (DEI) in the workplace. This not only is concerning for women and minorities who are looking for encouragement through mentors and examples of glass ceiling breakthroughs but also has serious implications in general for companies that need to become more diversified, rather than less, if they want to succeed.

Why, despite work over the past 10 years to promote DEI, are women stepping away from their companies instead of fighting for change? The report details that the interviewees felt they continue to face barriers that indicate it will be harder to advance than if they were male. They are more likely to experience something called “microaggressions,” such as

  • having their judgement questioned
  • being assumed to be a more junior manager
  • being accused of having achieved their senior rank only because they are a woman and not because they earned it.

Connecting the McKinsey Report to the DAC Panel Female Leaders

Panelists at the DAC event shared that they have faced many of the same issues mentioned in the McKinsey report. Radhika, group director at Synposys, has been with the company 20 years and commented that despite the belief that moving around can advance your career, what worked for her was finding a company that fit her needs, with passionate people and an open culture, and staying.

Susanna commented that when she joined a startup, all of the men had Ph.D.s, and she suffered from the typical behavior of not speaking up and never asking for anything. Most of the time, the men were not actively holding her back, but they were not supporting her either. She also believes that while the big things are important, sometimes the microaggressions are not worth making a fuss about, and it is harder to find someone to empathize.

Women are Demanding More (Not More Demanding)

In addition to gender barriers and perceived inability to advance as quickly as men, the report says that women are increasingly leaving their jobs as they want more flexibility, a better work/life balance, and DEI and therefore are leaving companies that do not support these ideals.

The report warns that if companies do not start taking these issues seriously, they risk losing not only the highly valuable and experienced women they already have but also the next generation of women leaders since young women entering the workforce are even more ambitious than their predecessors, while also expecting the flexibility and inclusion being demanded by their seniors. “[Young women] are watching senior women leave for better opportunities, and they’re prepared to do the same,” the report says.

The DAC panel discussed how to implement diversity. Susanna felt that quotas or a proportion of women has felt to her like to this day, people still say she only got her position because she is a woman. Radhika said women should not think it is someone else’s responsibility to fix. It is interesting that the women interviewed for the McKinsey report said they feel like they do the heavy lifting in supporting employee well-being, mentoring, and inclusion, yet this work, which takes a significant amount of time and energy, is largely unrecognized, unvalued, and unrewarded when it comes to performance reviews.

Conclusion: My Call to Action

I can personally recount numerous stories among the many women I have met over the years through my WIM activities and outreach who have left to pursue other firms perceived to be more supportive. The first person’s story I will share was a higher-up manager at a major software firm who decided to resign when she found out she was pregnant rather than deal with the career reset she believed she would face if she were to return after maternity leave. The next person’s story is one of a midlevel manager at a large test and measurement firm who opted to pivot out of the industry to pursue her passion elsewhere as she found more joy in starting over fresh than in continually advocating for herself without much additional support internally for her own career trajectory. And then most recently, I had dinner with an IEEE (female) fellow, who shared with me that she is burned out fighting day in and day out for equality and career advancement at her company and would welcome the support of any and all male advocates to pull her into new work challenges and even career advancement opportunities.

Unfortunately, these are not the only stories I can share. Yet, there is a positive message to come from these in that as managers, regardless of the genders on our teams, we must act and advocate for all employees and, in particular, be mindful of the women on our staff and overcome unconscious biases in whatever form they manifest.

My call to action to the readers of this column, both men and women, is to be an advocate for the small community of WIM, be aware of unconscious biases, and to not shy away from shining a spotlight on the WIM role models we do have so that they can continue to inspire the next generation of women in tech and eventual leaders. Make it your mission today to write the names of the amazing women you know on a sheet of paper and commit to taking action to advocate for them this year and next whether for a role within the IEEE Microwave Theory and Technology Society or your own employer (academia, industry, and/or government).

References

[1] D. Rock and P. Gercovitch. “Why diverse teams outperform homogeneous teams.” Neuroleadership.com. Accessed: May 24, 2023. [Online] . Available: https://tinyurl.com/jx5hwmjm

[2] D. Rock and H. Grant, “Why diverse teams are smarter,” Harvard Bus. Rev., Nov. 2016. Accessed: May 24, 2023. [Online] . Available: https://hbr.org/2016/11/why-diverse-teams-are-smarter

Digital Object Identifier 10.1109/MMM.2023.3294881

IEEE microwave magazine   October 2023

Title

IEEE Microwave Theory and Technology Society Microwave Measurements Technical Committee Report for 2021–2022

Antonio Raffo, Dominique Schreurs, Marc Vanden Bossche

IMAGE LICENSED BY INGRAM PUBLISHING

The mission of the IEEE Microwave Measurements Technical Committee (TC-3) of the Microwave Theory and Technology Society (MTT-S) is to disseminate the metrology culture all over the world. It is a culture of peace since it is based on a fundamental pillar: developing synergistically scientific and technological knowledge based on cooperation, interdisciplinarity, and inclusivity for achieving sustainable progress for the future of all people on Earth. As a matter of fact, the ancient roots of this culture are in the Metre Convention (1875), to which adhere 100 states (64 member states and 36 associate states and economies). In the microwave community, our members give continuous stimuli to the development of instrumentation and measurement techniques that represent the conditio sine qua non to properly design and accurately characterize RF, microwave, millimeter-wave, and terahertz devices, circuits, and systems. Nowadays, the need of advanced instrumentation and calibration techniques is becoming increasingly essential for supporting the most challenging and timely research topics, spanning from quantum computing, which crucially requires microwave measurements at cryogenic temperatures, to over-the-air multiple input, multiple output systems, where the number of signal paths and wireless operation critically entail innovative measurement hardware in combination with calibration and measurement procedures. The amazing potential and features of microwave instrumentation and measurement techniques allow opening new frontiers in an ever-growing spectrum of applications, among which one new frontier is, nowadays, represented by the biomedical and health care applications.

Dissemination Activities

The microwave instrumentation currently available, thanks to a high level of automation and an extremely simplified user interface, tends to hide the necessity of the deep knowledge required to perform accurate measurements at microwave through terahertz frequencies. As a general remark, it should be pointed out that in the expression of measurement uncertainty, the contribution related to the operator, which in most cases is the dominant one, is often completely neglected. Accurate measurements derive from the profound knowledge of the adopted instrumentation and of the measurand. Low-frequency operation instruments can be considered ideal, but this is clearly not true when the instrumentation is designed to put forward the state of the art using circuits at the limit of the available technologies. Such a consideration justifies our efforts to promote microwave metrology by sponsoring and organizing seven workshops in 2021–2022 in different research fields of the MTT-S community. In particular, the workshops were organized in the framework of the following conferences: 2021 IEEE Radio and Wireless Week (Modeling and Design Tools for Accelerated Design of 5G GaN PAs), the 2021 IEEE International Microwave Symposium (Calibrated Testbeds for the Characterization, Optimization and Linearization of Multi-Input Power Amplifiers, as well as Platforms, Trials, and Applications: The Next Step for 5G and Future Wireless Networks), the 2022 IEEE International Microwave Symposium (Emerging Low-Temperature/Cryogenic Microwave Techniques and Technologies for Quantum Information Processing; Measurement and Modeling of Trapping, Thermal Effects, and Reliability of GaN HEMT Microwave PA Technology; and On-Wafer mm-Wave Measurements), and the 2022 IEEE European Microwave Week (Microwave Design and Metrology for Quantum Computing, as well as Power and S-Parameter Measurements at Millimetre-Wave and Terahertz Frequencies: Recent Research Progress and Metrology Capabilities). We especially mention Prof. Patrick Roblin, who is the TC-3 workshop coordinator.

We are really proud of the continuous and prestigious activity of Dr. Jon Martens, as 2020–2022 Distinguished Microwave Lecturer (DML) (Figure 1). His talk “What Is My Measurement Equipment Actually Doing? Implications for 5G,” an amazing journey in instrument architectures considering the necessity of working at frequencies above 100 GHz and with wide modulation bandwidths, was one of the most requested by the MTT-S community. Another reason to be proud is the appointment of Prof. Nuno Borges Carvalho as MTT-S president. Concerning DML activities, we wish to thank Prof. José Carlos Pedro for his constant efforts in searching for valuable candidates: for the class of 2023–2025 DMLs, we endorsed the candidature of Prof. Joseph Bardin with the talk “Quantum Computing: What Is It, How Does It Work, and What Are the Opportunities for Microwave Engineers?”

Figure 1. Dr. Jon Martens, 2020–2022 DML.

Membership

TC-3 consists of 25 members (https://mtt.org/technical-committees/tc-3-microwave-measurements-committee/members/) from industry, national metrology institutes, and academia, including eight Young Professionals and three Speakers Bureau speakers. We especially thank our four affiliate members (Dr. Ricardo Figueiredo, Dr. Debapratim Ghosh, Dr. Gian Piero Gibiino, and Dr. Nosherwan Shoaib) for the energy and enthusiasm they gave generously in all of the activities they were involved in: from the initiatives for the 70th anniversary of MTT-S, to the organization of a student design competition (“Measurement and Extraction of Device Parameters of an RF Transistor”) in the framework of the 2022 IEEE International Microwave Symposium. A number of members retired from the committee during these two years; it is our pleasure to thank all of them for their invaluable contribution to TC-3 activities. In particular, we would like to recognize the scientific contributions to the microwave measurement research field of two innovative, extraordinary researchers: Prof. Andrea Ferrero and Prof. Yves Rolain.

Technical Contributions

TC-3 members have actively contributed to the advance of measurement and calibration techniques for all of the fields and applications of interest for MTT-S, enabling and boosting the development of entire research areas. During these last few years, TC members have also published extensively in high-ranked journals and at international conferences. Among the many activities, we would like to write a few words on one of the most important TC-3 contributions to MTT-S, i.e., our strong involvement in the development of standards. Many TC-3 members are involved in this activity, as both working group participants and chairs; we especially thank Dr. Nick Ridler and Dr. Andrej Rumiantsev, who coordinate these fundamental activities inside our committee. The standards in which TC-3 is actively participating are as follows:

  • P2822: “Microwave, Millimeter-Wave, and THz On-Wafer Calibrations, De-Embedding, and Measurements”
  • P1770: “Usage of Terms Commonly Employed in the Field of Large-Signal Vector Network Analysis”
  • P287: “Precision Coaxial Connectors at RF, Microwave, and Millimeter-Wave Frequencies”
  • P1765: “Estimating the Uncertainty in Error Vector Magnitude of Measured Digitally Modulated Signals for Wireless Communications”
  • P1785: “Rectangular Metallic Waveguides and Their Interfaces for Frequencies of 110 GHz and Above.”

Future Plans

In the coming two years, TC-3 wants to continue the impactful work of its members in line with the previously described contributions. However, RF measurement science needs to evolve, and new multidisciplinary characterization challenges need to be embraced as they present themselves today and in the near future. TC-3 wants to help the global industry to reduce or overcome these challenges.

The level of integration of semiconductor technology from digital to RF in combination with fast evolving packaging techniques leads to new innovative applications impacting our daily life as we communicate with each other, drive our cars, receive medical care, and more.

“Being connected everywhere at any time” in combination with different forms of sensing is constantly increasing the number of channels and number of states per channel in RF devices. Routing signals from instruments to these devices or vice versa on wafer or off wafer and still being confident in the measurement quality at ever higher frequencies and larger modulation bandwidths is not obvious, and the calibration techniques become tedious and very time consuming.

At the same time, the integration with antenna elements eliminates the “luxury” and convenience of a well-defined interface between the instrument and the device under test, namely, the TEM mode. Consequently, there will be an evolution from parametric testing to functional testing, which is typically very application specific. Emulating environmental conditions from a device, e.g., a transistor, to a system will be essential to minimize the time to market.

Through collaboration within TC-3 with academia and a closer interaction with industries who face these challenges, TC-3 wants to develop in the coming years best characterization practices and wants to help standardization, which enables the development of ecosystems in line with industrial needs. New calibration, correction, and measurement techniques will have to be developed in support of the functional testing while being as independent as possible from specific applications.

Digital Object Identifier 10.1109/MMM.2023.3294880

IEEE microwave magazine   October 2023

Title

Swapping Tales

Steve C. Cripps

IMAGE LICENSED BY INGRAM PUBLISHING

Now and again one sees, or even invents, something that could be termed a solution without a problem (SWAP). Curiously though, sometimes these SWAPs turn out to be not only useful after all but maybe even transformational. This probably applies to several of the familiar accoutrements of daily life in the 21st century: the electric car, the Internet, the personal computer, and more. Closer to the home of microwave technology, I remember the term SWAP being applied to microwave monolithic integrated circuits (MMICs) in their evolving era of the 1980s; it seemed that the amount of gallium arsenide required to implement the passive elements of a functional MMIC would always be much more expensive compared to the existing, ubiquitous chip-and-wire hybrid approach. The rest of the MMIC story, as they say, is indeed history.

However, not all such inventions lead to such a happy ending, and the path of “progress” is strewn with SWAPs that get quickly written out of the script. I rather think many of us have experienced the SWAP effect early in our careers and can still be heard, on occasions, boring the pants off our younger colleagues as we wax lyrical about them. That is exactly what I plan to do—so, with no further apologies, I present the avalanche diode and, most importantly, its rather mysterious and short-lived evolution, the “anomalous avalanche mode,” or TRAPATT, an unlikely acronym from “trapped plasma and avalanche-triggered transit” device.

Dedicated readers of this column, should there be any left, may remember that I did broach this subject some years ago [1], although this was something of a more formal recognition of the academic approach to the subject, most notably promoted by Carroll [2]. Indeed, this particular reference (Carroll, not Cripps—later my own Ph.D. advisor) was itself unorthodox inasmuch as it focused on the analysis of the TRAPATT circuit, rather than the device physics of the diode itself.

This was the pre-GaAsFET era, when microwave semiconductor research was all about two-terminal devices in the form of “transferred electron” or “Gunn” diodes, which were not actually diodes, and avalanche diodes, which were. The most familiar brand of microwave avalanche diode was the IMPATT (impact avalanche and transit time), which was able to produce oscillations at gigahertz frequencies and has, marginally, survived to the present day. Both the Gunn and the IMPATT were fundamentally oscillators in themselves; the basic semiconductor chip itself would oscillate under suitable bias conditions. Indeed, J.B. Gunn, the inventor of the Gunn diode, famously commented that the most important observation he had ever written down was the word “noisy,” in his notebook, when measuring the I–V characteristics of gallium arsenide samples. The IMPATT has a somewhat similar history, inasmuch as it was predicted as a theoretical concept well before the proposed structure could be fabricated [3], but oscillations were later observed experimentally and, fortuitously, using diodes that had not originally been fabricated for this purpose.

This serendipitous aspect of avalanche diode oscillation was certainly an important aspect of my own work on TRAPATTs. Two main features differentiated the TRAPATT from the more well-known IMPATT: the TRAPATT appeared to deliver much higher powers, one or even two orders of magnitude higher than either Gunn or IMPATT diodes of similar physical size, and, somewhat conveniently, showed comparably quite high efficiencies, in some cases approaching 50% at power levels approaching a kilowatt. The RF waveforms were characterized by very short current pulses, fewer than 100 ps, and a spikey voltage that collapsed rapidly after peaking well above the breakdown level. As such, most TRAPATT results were obtained using pulsed bias supplies running at low duty cycles between 0.1% and 1%. Here was the SWAP: the TRAPATT could deliver large powers, levels unheard of for a semiconductor device at the time and still “healthy” today, with very small, cheap structures—but only for a microsecond or so. The obvious application was radar, but the rather noisy spectral properties of the TRAPATT resulted in a fairly rapid decline of interest in this application, the radar system industry being more interested in the possibilities being strongly touted by MMIC developers for phased-array radar systems.

One of the interesting aspects of any SWAP narrative is to consider, or speculate about, what the impact of today’s technology would be. The TRAPATT era was pretty much confined to the 1970s, when solid-state microwave power technology was struggling to generate even a watt or two of power at gigahertz frequencies. Having myself built TRAPATT oscillators using cheap wire-ended computer diodes, in which the tiny die itself is essentially suspended in inside a small glass package but that could still generate 50 W of power for a few hundred nanoseconds before it fried, I have always wondered what could be achieved using modern fabrication techniques. For example, if the duty cycle could be increased and the RF pulse length substantially increased, other applications may start to come within range. However, to get any interest at all, it has always seemed that I will have to reinvent the TRAPATT myself, as one of the very few remaining TRAPATT practitioners still around and with access to the necessary equipment. Before I describe such an effort (yes . . . it’s coming.!), it would be worth backtracking a little to the essentially still unresolved questions of how the TRAPATT really works and, even more mysteriously, how it starts.

Pretty much all of published attempts to explain TRAPATT oscillation focus on the device itself and, in particular, the physics of avalanche breakdown. There is much talk of the Read equation [3], avalanche shock fronts, and trapped plasmas. The role of the passive circuit somehow gets secondary status; it was given little more than a handwave. I prefer to reverse that process to consider a typical TRAPATT circuit and show, with my own handwave, how it is at least conceivable that large signal oscillation could be sustained by a diode driven repeatedly and quickly into its breakdown regime.

Figure 1 shows an idealized set of TRAPATT diode waveforms, as predicted and—rather less frequently—actually measured. The key aspect of avalanche breakdown is the dynamic behavior when viewed on picosecond timescales. The current waveform is a sequence of very short pulses, which are generated due to the voltage, which sweeps to a peak value well above the avalanche breakdown level and then collapses due to the impedance of the external passive circuit. The key feature is that the avalanche current multiplication continues while the voltage is above the breakdown level, even though it has passed a peak value. In retrospect, looking at the idealized waveforms in Figure 1, it is obvious that the voltage closely resembles the derivative of the current, with suitable scaling and offset. This is certainly a handwave, but there certainly seems to be the possibility of continuous oscillation once the process has been initiated if the external circuit can act essentially as a differentiator—the trick being to generate enough charge in each cycle for the circuit to produce the necessary overvoltage kick that keeps the process going.

Figure 1. Idealized TRAPATT waveforms. LPF: low-pass filter.

However, how such an oscillation gets started has remained something of a mystery that was never fully solved. Gigahertz-bandwidth oscilloscopes in the 1960s era were entirely of the sequential sampling type, and, although TRAPATT waveforms were reported, looking remarkably similar to those in Figure 1, such an instrument was not capable of displaying the very noisy oscillation buildup period.

Most reported TRAPATT circuits had the basic form shown in Figure 2. The diode is placed at the end of a length of transmission line, which initially is considered to be terminated with a short circuit. A key observation is that the TRAPATT oscillation frequency is always slightly higher than the half-wavelength of the line, so that, at the oscillation frequency, the line length is slightly shorter than a half-wavelength. I will not repeat the analysis of Carroll [2], having already done so in [1], which shows that, if the shortfall is small enough, and the frequency analysis is restricted to harmonics of the fundamental only, such a circuit behaves as a negative inductance, thus providing the required differential voltage. Needless to say, and in the serendipitous spirit of the TRAPATT, this circuit was demonstrated well before the theory was published, but looking at the “Evans” TRAPATT circuit on a modern simulator certainly upholds the “differentiator” function and suggests that various other circuit topologies may well work.

Figure 2. A TRAPATT oscillator circuit.

If we assume that the diode delivers a short repetitive pulse into this impedance, being inductive, the voltage across the diode will be a scaled derivative of the current, which is offset by the dc supply, as indicated in Figure 1. Therefore, if the dc supply is set to a level just below the diode breakdown, the voltage will swing the diode briefly into its breakdown region, and carrier multiplication will escalate rapidly and only then to be suppressed when the voltage collapses due to the differential action of the circuit. Therefore, continuous oscillation is conceivable; a few tweaks in the various parameters that characterize the avalanche breakdown and, especially, how the generated charge is extracted from the junction after the voltage collapses are necessary to convert a handwave into reality—but sometimes, it does all appear to work .

As such, I certainly do not dismiss the focus on the device physics in the vast majority of papers and articles published on TRAPATTS in the 1970s, although, with this long in retrospect, it is clear that, in devising suitable diode doping profiles, it was clearly quite easy to come up with structures that had the “necessary” properties, while experimental evidence suggested that “sufficient” properties could also be demonstrated using diodes that were never intended to work at gigahertz frequencies at all, never mind to generate tens or hundreds of watts of power. Custom or otherwise, TRAPATT oscillators that yielded tens of watts at efficiencies approaching 50% were reported at frequencies up to X-band and hundreds of watts were reported at lower gigahertz frequencies.

The first indication of the serendipitous TRAPATT was in a short paper [4] that described a cheap commercial diode, the Fairchild FD300, as working in a TRAPATT circuit. Admittedly, the impressive power of 68 W was obtained at a somewhat low frequency, by today’s standards, of 630 MHz, not to mention a very short pulse and long duty cycle, but it did rather set a cat among the pigeons as far as the TRAPATT theories were concerned. It also opened up a door for doing some research into TRAPATTs without necessarily having access to a semiconductor fab (as they were not then known) to manufacture custom diodes. I was therefore somewhat fortunate, depending on one’s views on SWAPs, to find this open door as I started my Ph.D. work.

Curiously, one reason why playing the TRAPATT game—essentially, testing any diode that came to hand for its potential TRAPATT properties—was not a widespread activity was not so much due to the design and construction of the oscillator circuit, which were remarkably simple but to the difficulty of finding a suitable generator for the bias pulses. At that time, generating reasonably sharp 1-µs pulses of up to 200 V at currents of 1 or 2 A was well outside the capability of regular lab pulse generators, as it still is. Commercial products were available, such as a classical product from Velonex, but more Heath Robinson contraptions involving a long length of coaxial cable and a mercury-wetted relay were quite commonly employed.

At the time, I did, in fact, have access to a Velonex pulser, which was full of ultrahigh-frequency vacuum tetrodes and could deliver 1-kV pulses with a risetime of about 10 ns. This was considered important inasmuch as no one seemed to have much of a theory as to how the TRAPATT oscillation started. Being very much a large signal effect, it was not possible to see how it could build up gradually from the noise floor, and one possibility was that the initial kick of the bias pulse may get things started. This is certainly an area where a half century of development in power electronics can make a huge difference; such bias pulses can now be generated quite easily using a high-side PMOS switch, and it was an awareness of this that made me speculate about whether I could repeat some of the TRAPATT results reported in the 1970s. This was only in part a nostalgic exercise; recent work on the interactions of microwaves with biological samples have shown some interesting results such a “electroporation” of cells [6], and the use of short pulses is a way of excluding local heating effects.

The 1S44 diode had already been established as one that readily performed as a TRAPATT oscillator at low gigahertz frequencies, albeit with somewhat lower power and efficiency than the custom diodes being reported in the literature. It was generally described by the then-manufacturer as a “core driver” diode, used in ferrite core memory banks. I also managed, with statutory serendipity, to discover the 1S952, also a core driver diode, which had higher breakdown and improved TRAPATT properties [5]. Fifty years on, a quick search on eBay did not reveal any 1S952s, but I did obtain a batch of 1S44s (Figure 3). Whether these were “original” or a modern equivalent I had no idea, but the project appeared to have been launched, and I had to take a YouTube lesson on the design of a high-side switch, which quickly resulted in a viable circuit that would handle 200 V and a couple of amps while delivering a pulse of a few hundred nanoseconds.

Figure 3. 1S44 diodes.

Although a TRAPATT circuit appears very simple in concept, being essentially a length of transmission line terminated in a short circuit, there are a couple of extra issues to consider. The waveforms clearly contain substantial higher harmonic components, and the line has to be able to support these without much loss. It is also necessary to consider how to extract power from the oscillator. This was achieved by replacing the short with a low-pass filter that reflected harmonics but introduced an antiphase voltage component at the fundamental [2].

Therefore, armed with this rather ragtag collection of memories and handwaving theories, I cobbled up the test circuit shown in Figure 4. It is, simply, a microstrip line terminated at one end by the diode, and the other end is a low-pass filter, which was rather crudely designed to match the diode impedance at a predicted oscillation frequency around 2.4 GHz. Setting the pulsed bias supply to give about a 0.5-µs pulse at very low duty cycle (1-kHz pulse-repetition frequency) and with a curious mixture of nostalgia and anticipation, I gradually turned up the voltage to the point where breakdown commenced. Sure enough, and, admittedly, following some hours of circuit tweaks and diode burnouts, I obtained the bias voltage waveform shown in Figure 5, which displays a classical signature of oscillation whereby, after an oscillation buildup period, the mean diode voltage drops to a level substantially below the breakdown voltage. This indicates that there is an RF oscillation, which swings the voltage above and well below the breakdown level, thus generating sharp pulses of current, probably, in this case, in the region of a 10-A peak. The second trace in Figure 5 shows the detected 2.4-GHz RF output, which, after some judicious tuning and bias adjustments, indicated a pulse power of about 30 W.

Figure 4. The TRAPATT evaluation circuit board.

Figure 5. The TRAPATT voltage bias (lower trace, 50 V/div) and detected RF (upper trace, approximately 20 W/div; horizontal: 80 ns/div).

One has to be cautious indulging in technical nostalgia, but this still remains, 50 years on, the most remarkable result I have personally experienced. Take a small, cheap computer switching diode, drop it into a simple circuit, and get 30 W of microwave power. Drinks on me tonight.

There are caveats. As indicated in Figure 5, the oscillation takes many RF cycles to become established, and this very noisy start-up was one of the reasons the TRAPATT had such a short attention span. This made it difficult to display anything approximating an RF waveform. Sampling oscilloscopes available at that time typically had sampling rates no higher than 100 kHz, so, for a bias pulse length of less than a microsecond, each point on the RF waveform had to be sampled from successive bias pulses. Therefore, in devising a suitable triggering scheme, it was important only to trigger the sampling process once the oscillation had stabilized, and this required some trickery [7]. The design of suitable voltage and current probes was also challenging, and this is a subject I have addressed in a previous “Microwave Bytes” article [8].

All of that said, I cannot resist the temptation to show my best effort in capturing the RF voltage, shown in Figure 6. The calibration of the probe has to be at best approximate and is certainly questionable, but the general form of the voltage does appear to confirm TRAPATT oscillation, noting that the basic reactive waveform in Figure 1 now has an additional fundamental sinusoidal component due to the power extraction.

Figure 6. The measured TRAPATT diode RF voltage waveform. (See the text regarding probe calibration.)

So that’s about it—an illustrated history of a SWAP that never went anywhere further. “Big deal,” I hear the “gallium nitride (GaN) generation” saying. “Just 30 W and only for a couple of hundred nanoseconds?!” The key point, however, is that the active device here is a tiny, simple little silicon diode, probably costing a few cents if it were in production today. The short pulse length is entirely a function of the package, which provides essentially no heat sinking at all. For some decades, I have felt that modern microwave semiconductor fabrication could surely come up with a TRAPATT diode capable of delivering hundreds of watts for tens of microseconds. More speculatively, I wonder whether some emerging industrial microwave applications really need the pristine signal generated by a GaN transistor, which is required for telecom applications but seems something of an overkill when only raw microwave power is required. The TRAPATT does open a door that reveals the avalanche breakdown effect as an alternative simple and cheap way of generating large amounts of microwave power, limited only by thermal considerations.

Maybe the “Avalatron” could yet replace the magnetron .

References

[1] S. C. Cripps, “Trapwave Inc. [Microwave Bytes] ,” IEEE Microw. Mag., vol. 9, no. 4, pp. 46–51, Aug. 2008, doi: 10.1109/MMM.2008.924789.

[2] J. E. Carroll, “An analytic theory for the Evans circuit for avalanche diodes,” IEEE Trans. Microw. Theory Techn., vol. 18, no. 11, pp. 977–979, Nov. 1970, doi: 10.1109/TMTT.1970.1127382.

[3] W. T. Read, “A proposed high-frequency, negative-resistance diode,” Bell Syst. Tech. J., vol. 37, no. 2, pp. 401–446, Mar. 1958, doi: 10.1002/j.1538-7305.1958.tb01527.x.

[4] R. J. Chaffin et al., “A poor man’s TRAPATT oscillator,” Proc. IEEE, vol. 58, no. 1, pp. 173–174, Jan. 1970, doi: 10.1109/PROC.1970.7579.

[5] C. Oxley et al., “Design and performance of I-band (8-10-Ghz) TRAPATT diodes and amplifiers,” IEEE Trans. Microw. Theory Techn., vol. 27, no. 5, pp. 463–471, May 1979, doi: 10.1109/TMTT.1979.1129650.

[6] S. C. Cripps et al., “A theoretical and experimental study of the antiparallel TRAPATT diode oscillator circuit,” in Proc. 3rd Eur. Microw. Conf., Brussels, Belgium, 1973, pp. 1–4.

[7] N. A. Slaymaker and J. E. Carroll, “Improved sampling technique for the observation of high harmonics in TRAPATT waveforms,” Electron. Lett., vol. 7, no. 18, pp. 554–555, Sep. 1971, doi: 10.1049/el:19710374.

[8] S. C. Cripps, “Probing times [Microwave Bytes] ,” IEEE Microw. Mag., vol. 10, no. 1, pp. 28–34, Feb. 2009, doi: 10.1109/MMM.2008.930689.

Digital Object Identifier 10.1109/MMM.2023.3294879

IEEE microwave magazine   October 2023

Title

OTA Testing of Electronically Scanned Antenna Arrays

Jia-Chi Samuel Chieh, Satish K. Sharma, Raif Farkouh, Sanghamitro Das, Everly Yeo, Maxwell Kerber, Randall Olsen, Emanuel Merulla, Stephen Badger

IMAGE LICENSED BY INGRAM PUBLISHING

Phased arrays in the last decade have made tremendous progress, mostly as a result of the maturity of silicon beamforming chipsets. With 5G communication links on the horizon, silicon beamformers have been front and center and commercial development has been accelerated, and as a result price economies have scaled [1]. System-level considerations are now being made, specifically regarding the level of integration [2], [3], [4], [5], [6], [7]. There have been many papers in the literature which document various high-performance phased arrays. Some notable published papers on this topic include [8], which presents a dual-band multistandard 5G millimeter-wave phased array; [9], an area-efficient 5G millimeter-wave transceiver front end; [10], a K-band hybrid packaged phased-array receiver with integrated antenna array; [11], a 33.5–37.5 GHz beamforming transceiver with hybrid architecture phase shifters; [12], a wide-band transmit and receive phased array which operates from 16 to 52 GHz; [13], which presents a silicon beamforming chip which can support C-, X-, Ku-, and Ka-bands; [14], a dual-polarized transmit phased array for a common data link; [15], a 1024-element satellite communication receive-only phased array; [16] and [17], which present a wafer-scaled phased array that operates in the G-band; and finally, [18], which presents a D-band submillimeter wave beamforming transceiver in silicon germanium (SiGe). Much of the focus of these papers has been on radio-frequency integrated circuit (RFIC) development, where silicon and SiGe afford the ability to support multiple channels and therefore tighter integration, as well as nuanced design and integration of the beamformer with a printed circuit board (PCB) antenna array. However, only a few papers describe the necessary steps, as well as the equipment needed, to do a line-of-sight (LOS), over-the-air (OTA) microwave link using digitally modulated signals. This is, after all, the final use case for these types of electronically scanned antenna arrays, the ability to close LOS microwave links.

In our previous work [2], we described the design and development of a 64-element transmit and receive (T/R), dual-slant polarized Ka-band phased-array antenna using silicon beamforming chips. Here, we leverage that phased array to demonstrate its OTA data throughput capability. A short distance (0.4 km) data throughput link was tested in [19], where a relatively lower data rate of 30 Mb/s was achieved using a 64-element dual-slant polarized Ku-band phased-array antenna. In this work, we have carried out the data throughput testing with the Ka-band design for a much longer 1.0 km OTA link with data rates as high as 150 Mb/s. The ability to characterize the link gives us insight into the operation of our phased-array antennas; it also allows us to extrapolate the maximum range which can be afforded by these phased-array antennas. We discuss the hardware setup, as well as how OTA testing is performed. “The Phased-Array Antenna Development” section gives details of the silicon beamformer that we used, as well as design insight into the phased-array antenna, which is realized on a multilayer PCB. The “Phased-Array Antenna Characterization” section describes indoor laboratory characterization of the performance of the phased-array. The “Over-The-Air Testing: Outdoor Measurement Results” section describes details of the OTA testing, which was performed at a 1.0-km distance.

Phased-Array Antenna Development

Silicon Beamforming Chipset

Today’s emerging 5G silicon RFICs can enable an extremely low-profile phased-array antenna design. The fully integrated chipset eliminates the need for discrete transceiver blocks and includes a polarization switch, a T/R switch, a low-noise amplifier, a power amplifier, phase shifters, and variable attenuators. The fully integrated chipset reduces overall size, cost, and RF losses. One of the commercial RFICs used for our Ka-band design is the Anokiwave AWMF-0116 [15], whose system-level schematic is shown in Figure 1. This chipset is a single-channel T/R chip that can support dual polarizations and operates between 26 and 30 GHz. The transmit channel has 20 dB of gain, with an OP1dB of 13 dBm. The receive channel exhibits 23 dB of gain, with a noise figure (NF) of 5 dB. Both transmit and receive paths have 6-bit control over both amplitude/attenuators. The chipset also has 6-bit phase shifters (average root-mean-square (RMS) phase error of 5°). This chip uses a double-pole double-throw switch to support dual polarizations. The chip consumes 350 mW on transmit and 250 mW on receive. It should be noted that the AWMF-0116 is no longer recommended for new designs. Table 1 shows a limited sampling of commercially available silicon beamforming chips that operate in the Ka-band. As can be seen, as RF beamformers have progressed, the number of channels a chip can support has increased, reducing the level of complexity required on the phased-array PCB. Additionally, newer generations of RF silicon beamforming chips allow greater output power and lower NFs. This allows phased-array systems to achieve higher effective isotropic radiated power (EIRP) and a lower antenna gain-to-noise temperature (G/T) from a given N-element array.

Figure 1. Single-channel system-level block diagram including the Anokiwave AWMF-0116.

Table 1. Limited sampling of commercial silicon beamforming chips in the Ka-Band.

Multilayer RF PCB

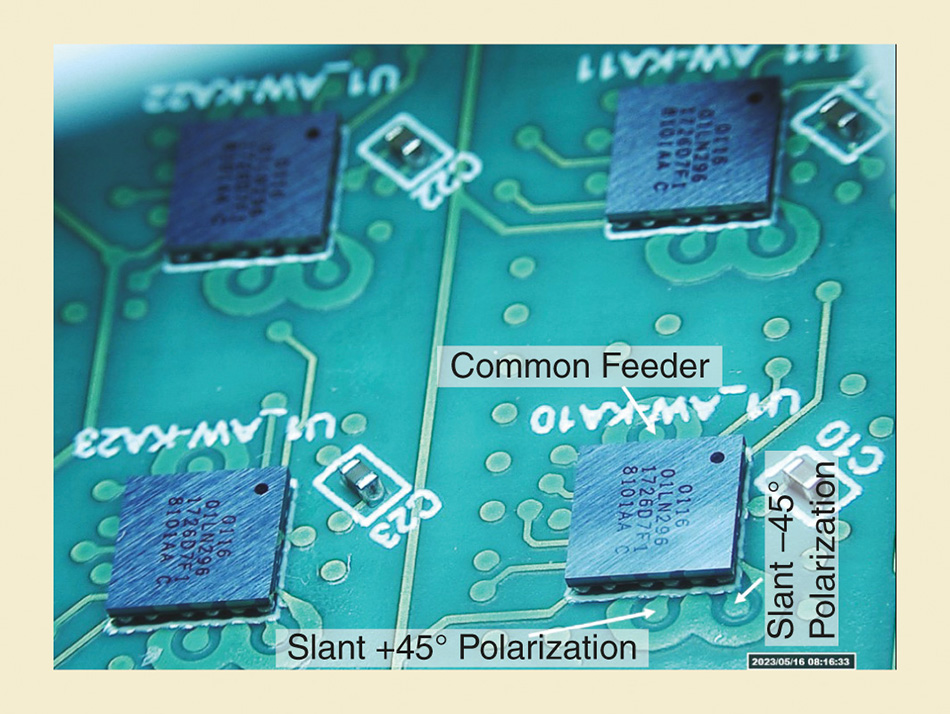
The top and bottom views of a fabricated prototype of the phased array are shown in Figures 2 and 3, respectively. Figure 4 shows a micrograph image of how the RF beamformers are attached to the PCB. As can be seen, the AWMF-0116 has wafer-level chip-scale packaging (WL-CSP) and a flip-chip attachment is used with fine-pitch solder balls. Since this beamformer supports dual-polarized antennas, we use finite-ground coplanar waveguide (FGCPW) transmission lines with a probe/via antenna feed. The FGCPW has ground vias which are used to improve the isolation between antenna ports. Some significant improvements were made to the phased array presented in this article. The improvements include optimizing the RF transition from the stripline feeder to the 2.92-mm coaxial connector, which was found to have significant mismatch loss in the original design. The pitch between ground stitching vias for the stripline feeder was greatly relaxed to enhance manufacturability and yield. Locking power and serial-peripheral-interface (SPI) connectors were implemented to enhance the resiliency of the interconnects during test events. Most significantly, a 65th RFIC was also added to the beamforming network board (Figure 3) as a preamp (Tx) and postamp (Rx) gain stage to compensate for the loss of the feeder network. This article also expands on details, including transmit characteristics, and reports on utilizing the phased-array antenna in an OTA demonstration. The total size of the PCB, including auxiliary connectors, is 3.5 in × 4.5 in (88.9 mm × 114.3 mm). To support low-cost solutions, a planar antenna integrated onto the circuit board is preferable. Figure 5 shows the multilayer PCB stackup. The antennas reside on a Rogers 4350 substrate, with 10 mils (0.25 mm) between the upper and lower patch elements. The antennas are probe-fed (50 Ω) with two vias with a diameter of 8 mils (0.2 mm), one for the slant +45° polarization, and the other for the slant −45° polarization. To enhance the bandwidth as well as support dual polarizations, a stacked-patch antenna was selected. Readers are encouraged to see articles [26], [27], [28], [29] for details related to the stacked-patch antennas and [30], [31], for circularly polarized variants of this type of antenna topology. The driven patch is 90 mil x 90 mil, and the parasitic patch is 95 mil x 95 mil. Finite array simulations were completed in Ansys HFSS [32] using the domain decomposition method, as shown in Figure 6. This unit cell approach allows for reduced computational times, while accounting for mutual coupling and edge effects. The array has an interelemental spacing of approximately 0.6${\lambda}$ at 28 GHz. The 8x8 array utilizes a stripline corporate feed network with Wilkinson combiners. Embedded NiCr thin-film resistors were utilized because the feed network resides between dielectric layers. Via fencing was implemented on the stripline layer to ensure the proper characteristic impedance of the transmission lines. The simulated excess feeder loss is approximately 14 dB. Figure 7 shows the PCB layout of the bottom layer where the RF beamformers reside, as well as the internal stripline layer. Care was taken to ensure that equal path length matching was achieved.

Figure 2. Top view of the generation 2 Ka-band 64-element T/R phased-array antenna.

Figure 3. Bottom view of the generation 2 Ka-band 64-element T/R phased-array antenna.

Figure 4. AWMF-0116 WL-CSP on the PCB.

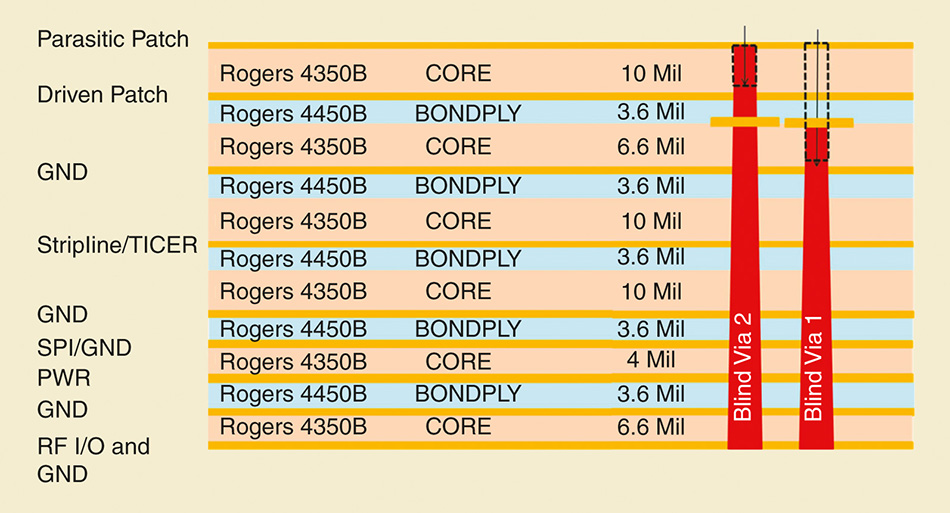
Figure 5. PCB stackup (10 mil = 0.25 mm).

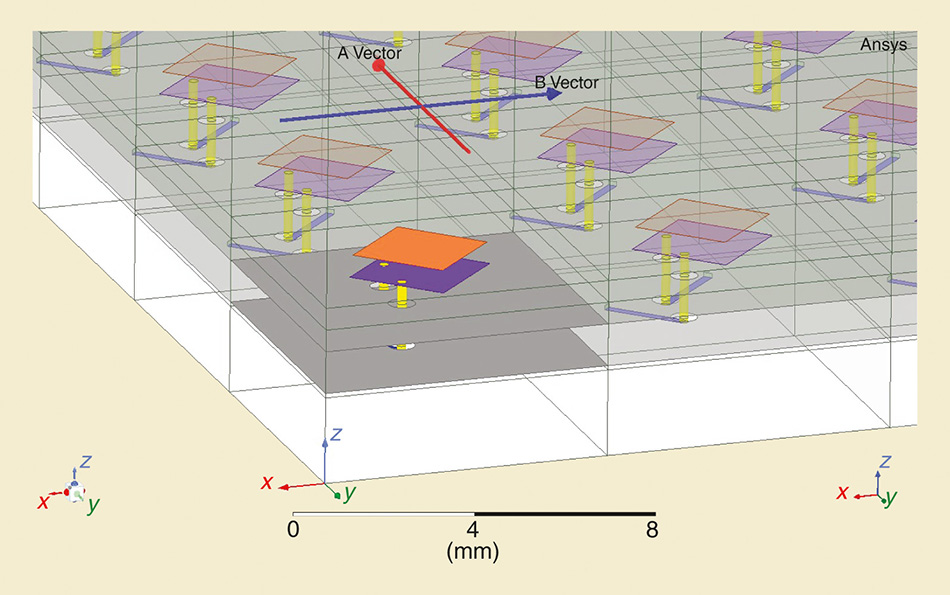
Figure 6. Finite-array analysis using HFSS domain decomposition.

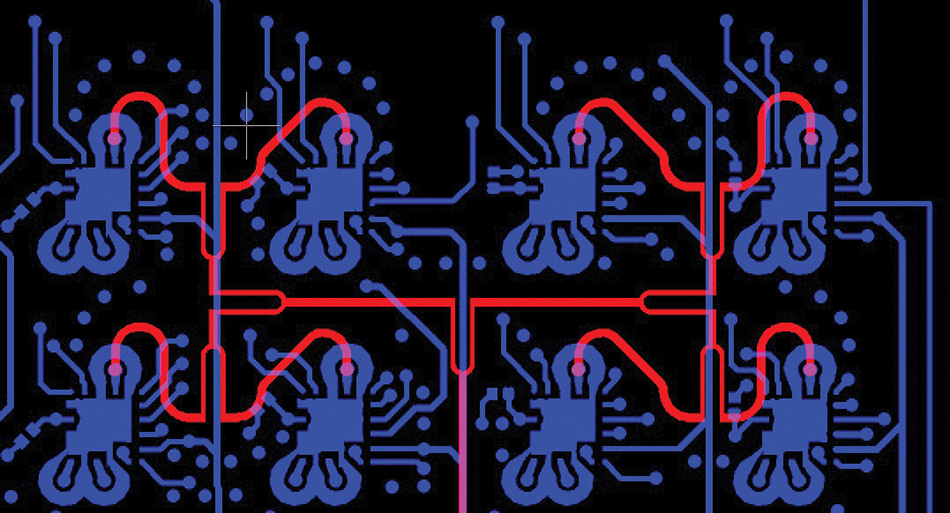
Figure 7. Stripline Wilkinson corporate feed network for a 2 × 4 subarray within the PCB.

Phased-Array Antenna Characterization

The AWMF-0116 chipset utilizes a five-wire SPI for applying the beamforming algorithm and controlling the array. A custom LabVIEW graphical user interface was written to control the array along with a National Instruments USB-8452 providing the SPI outputs.

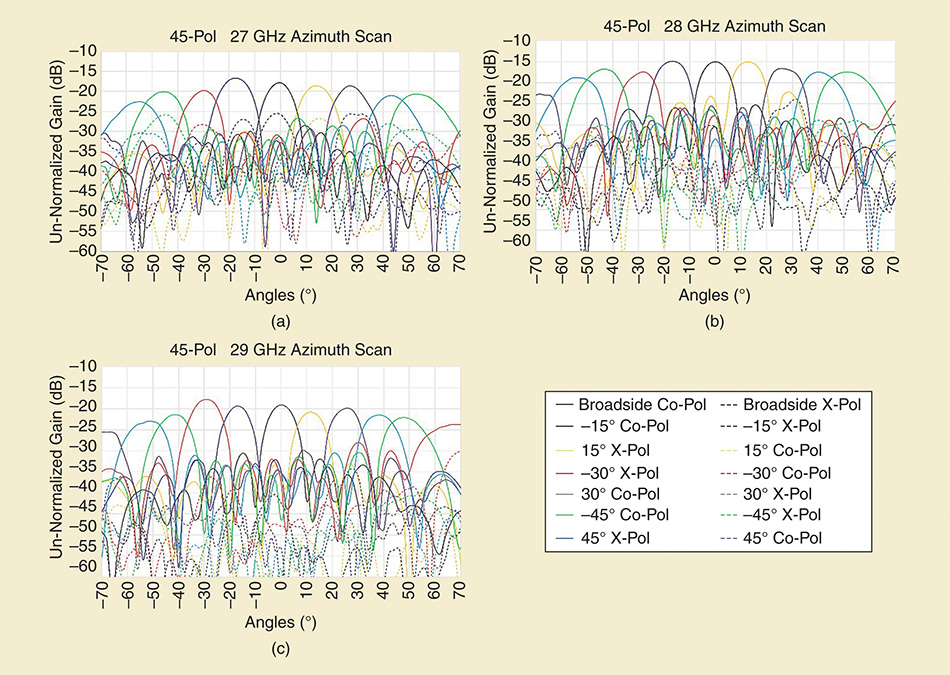
Since the phased-array antenna represents an improvement over the one presented in [2], we performed measurements in a far-field anechoic chamber at San Diego State University’s Antenna and Microwave Lab. Measurements were made from 27 GHz to 29 GHz for both polarizations and for both azimuth and elevation planes. For brevity, azimuth scan patterns from the +45° slant linear polarization and elevation scan patterns from the −45° slant linear polarization are presented. No phase or amplitude calibrations are applied, and as can be seen, the sidelobe levels are nearly equal. For larger arrays, calibration is important to ensure adherence to radiation pattern masks for various standards such as the 3rd Generation Partnership Project. Phased-array calibration is typically done element by element, where a single channel/antenna is turned on, and the amplitude and phase characteristics are measured. Once this is done across the entire array, amplitude and phase offsets can be introduced to ensure minimal phase and amplitude variation across the array aperture. Figure 8 shows the measured scan patterns of the array on the azimuth plane for the +45° slant linear polarization at 27 GHz, 28 GHz, and 29 GHz. As can be seen, the measured cross-polarization levels are well below 15 dB. Figure 9 shows the measured scan patterns of the array on plane for the −45° slant linear polarization, and also shows cross-polarization levels below 15 dB. Methods such as subarray mirrored symmetry [33] can further reduce the cross-polarization levels. The copolarization patterns have symmetric sidelobes, indicating that the standard deviation is tight from chip to chip for both amplitude and phase. The 64-element array can perform without a cold plate and without exceeding temperature limits. With the addition of a cold plate, the temperature can be reduced accordingly.

Figure 8. Slant +45° radiation patterns at (a) 27 GHz, (b) 28 GHz, and (c) 29 GHz.

Figure 9. Slant −45° radiation patterns at (a) 27 GHz, (b) 28 GHz, and (c) 29 GHz.

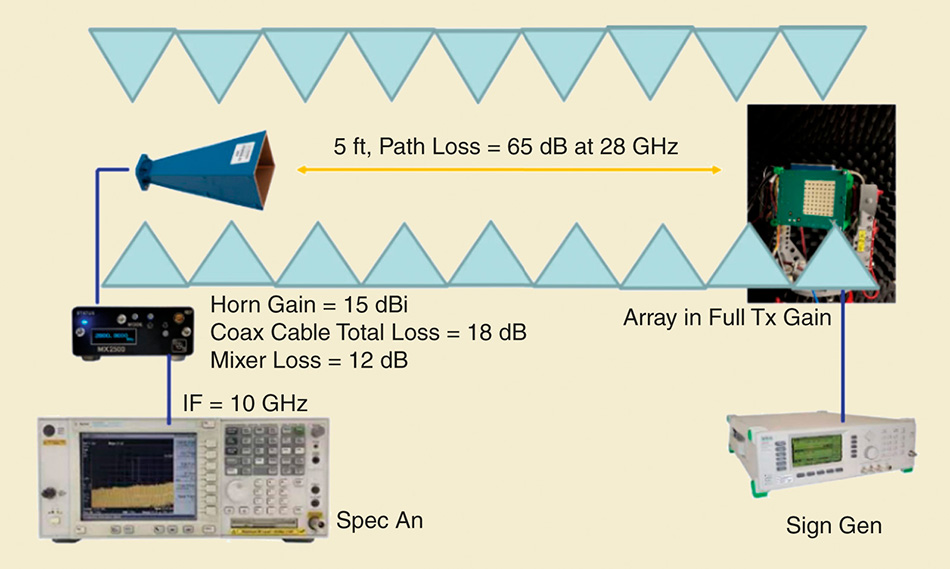
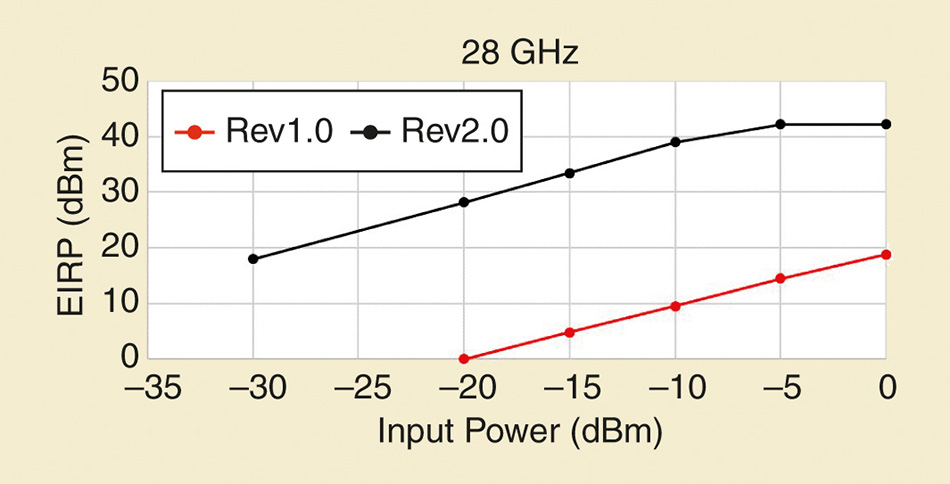
To test the transmit characteristics, we performed EIRP measurements in our test lab. This measurement is similar to measuring the compression point on a power amplifier, where the input power is slowly swept until the amplifier shows compression or saturation. However, in the EIRP measurement, as the input power is slowly swept from low to high values at the input of the array, the EIRP we are measuring is the combination of the aggregation of the power amplifiers within each of the 64 beamforming chips, as well as the gain of the antenna array. We performed this measurement inside our laboratory, with modular absorber foam walls to mitigate any reflections within our laboratory. A Wiltron 68369B was used as a transmit signal generator set at a frequency of 28 GHz. The signal generator fed the phased array directly, which was set to transmit mode. Approximately 5 ft (1.52 m) across the laboratory we had a Pasternack standard gain horn antenna, which was connected to a down converting mixer and then to the Agilent E4440A spectrum analyzer. This is shown in Figure 10. The phased-array antenna was set to maximum gain. The input signal was slowly swept from −30 dBm to 0 dBm at 28 GHz. Since we know the input power to the array, the path loss from the distance between the Tx and Rx antennas, losses from the test cables and external mixer, as well as the gain of the standard gain horn used on the receive side, we can therefore deduce the EIRP out of our phased-array antenna. Figure 11 shows the measured EIRP compression for this array, as well as a comparison to the array from [2]. Much like in power amplifiers, where the signal or waveform is typically operated at a 3–6 dB back off from compression to preserve linearity, the same can be applied to the phased-array antenna in transmit mode.

Figure 10. Laboratory test setup for measuring EIRP and Tx Compression (5 ft or 1.52 m).

Figure 11. Measured output 1-dB compression point of the phased array at 28 GHz.

As can be seen, the addition of the 65th RFIC compensates for the stripline feeder loss and allows a lower input power to be applied at the input of the array. This is practical because most commercially available radios that operate in the L-band (1–2 GHz) have output powers below 0 dBm. Since the 65th RFIC is placed immediately after the coaxial connector, rather than more strategically in the RF signal path, this 65th RFIC compresses before the 64-element level beamforming chips. This is the reason why the EIRP compresses at around 42.2 dBm. The architecture of the array was completed in this way primarily because of space constraints on the PCB, since the lattice spacing is small in the millimeter-wave frequency regime.

OTA Testing: Outdoor Measurement Results

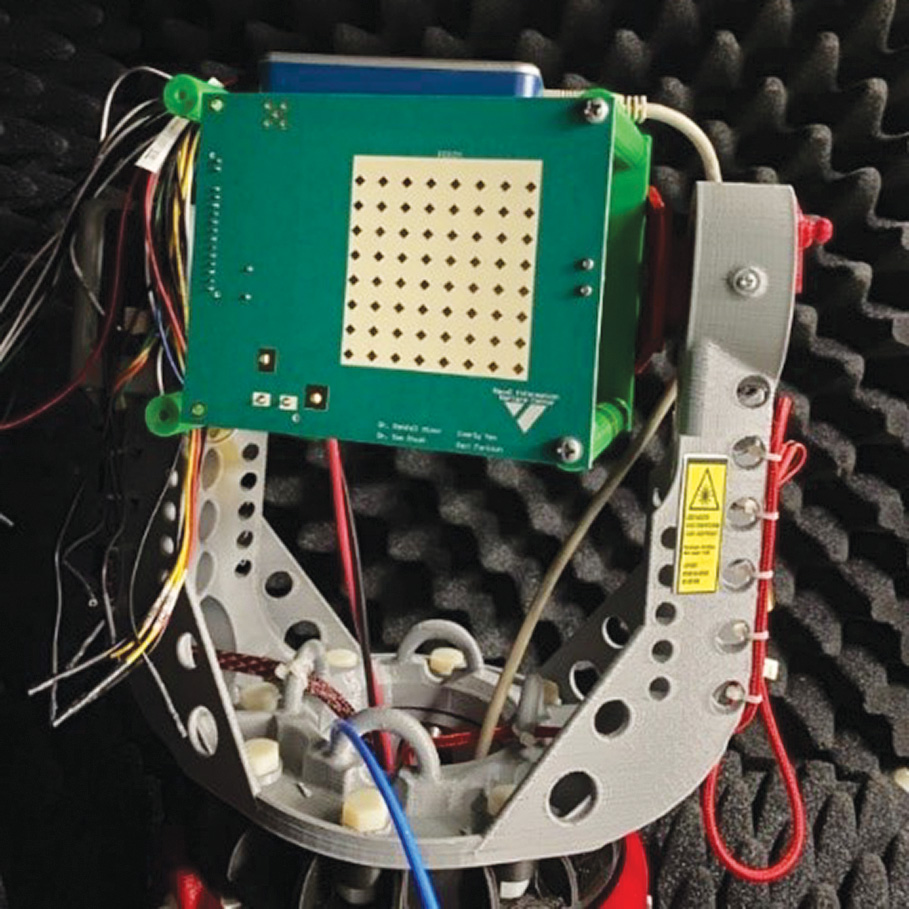
To characterize the performance of our phased-array antenna, we performed an OTA test at San Diego State University. There are very few reports in the literature which demonstrate a millimeter-wave phased array over long distance; rather, most instances are demonstrated over short ranges, such as in [34], which demonstrated a 50 m OTA link. The outdoor range for our test was approximately 1.0 km, and the two test sites were at the same altitudes for easy line of sight. The outdoor range is shown in Figure 12. The receive test setup is shown in Figure 13.

Figure 12. OTA 1.0 km test range at San Diego State University between the Physics Building and the Chapultepec Dormitory.

Figure 13. Test setup on Chapultepec Dormitory, Rx phased-array antenna.

To mimic the phased array and radio configuration in our outdoor testing, we utilized frequency converters. We used DSI Instruments MX30000 for the up/down converters. This mixer comes in a small form factor with an integrated frequency synthesizer which can be programmed through a USB connection. The local oscillator can be tuned from 18 GHz to 30 GHz. The insertion loss of the mixer is measured to be 12 dB at 28 GHz. A Keysight signal generator was used as a proxy for the transmitter, and a spectrum analyzer (Agilent E4440A) was used as a proxy for the receiver. Keysight VSA software was used with the E4440A to recover the constellation diagrams of the digitally modulated signals. Our measurement setup was limited by the instantaneous bandwidth of the signal generator, which was 75 MHz. The transmit frequency was set to 1.8 GHz, which is up-converted to 28 GHz over the air.

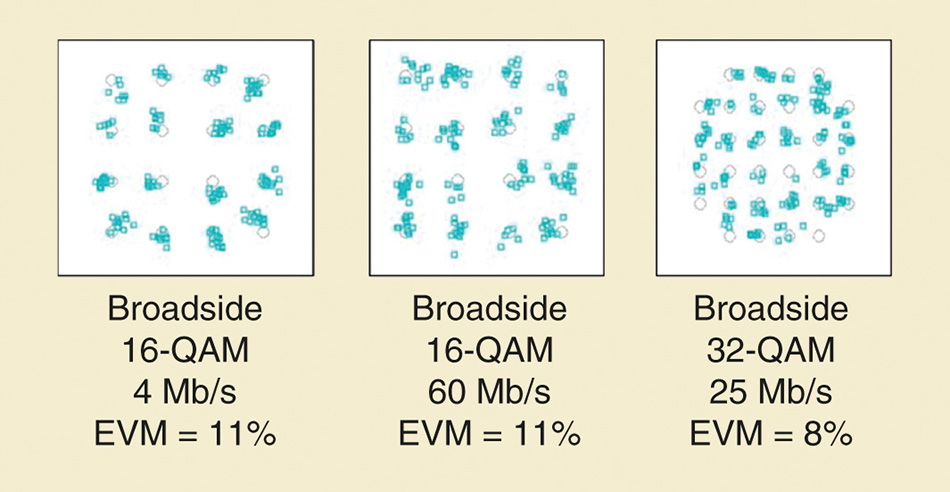
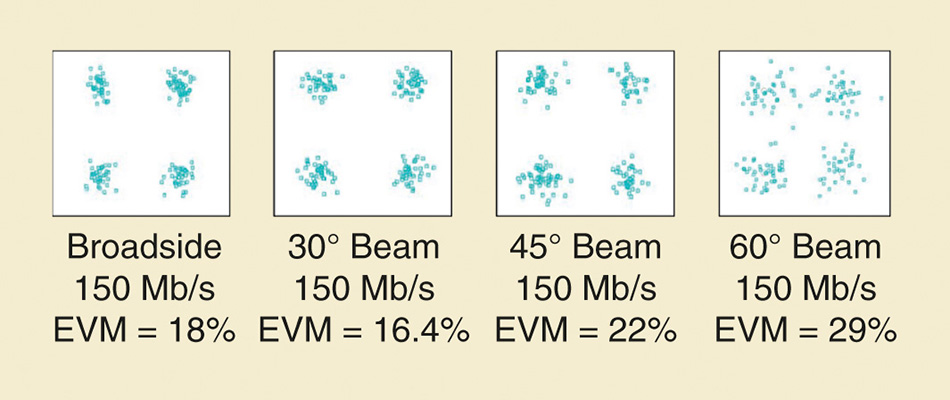
Phased-array antennas are used on both sides of the link. Figure 14 shows the recovered constellation of quadrature phase shift keying (QPSK) signals at 2–20 Mb/s, and 8-PSK (phase shift keying) signals at 3–60 Mb/s, with a broadside beam on both the Tx and Rx arrays. The resulting error vector magnitude (EVM) of the digitally modulated signal varied from 8% to 13%. Figure 15 shows the recovered constellations of 16-QAM (quadrature amplitude modulation) and 32-QAM signals from 4 to 60 Mb/s, showing a maximum RMS EVM of 11%. Recovering the digitally modulated signal over various electronically scanned beam angles is one of the advantages of using a phased-array antenna. For this test, the transmit phased array was set to various beam angles from 0° to 60°, in 15° steps. The transmit antenna was physically pivoted to offset the scan angle. Figure 16 shows the recovered constellation over various scan angles (0°, 30°, 45°, and 60°) with a QPSK modulation with a 75-MHz bandwidth. As can be seen, as the beam angle is increased, the EVM also increases. As the beam is scanned up to 45°, the RMS EVM is below 22%. At wide scan angles such as 60°, the gain of the array rolls off, and thus the SNR degrades, causing a higher EVM.

Figure 14. Recovered constellations for various bandwidths and modulations.

Figure 15. Recovered constellations for 16-QAM and 32-QAM.

Figure 16. Recovered constellations for various scan angles.

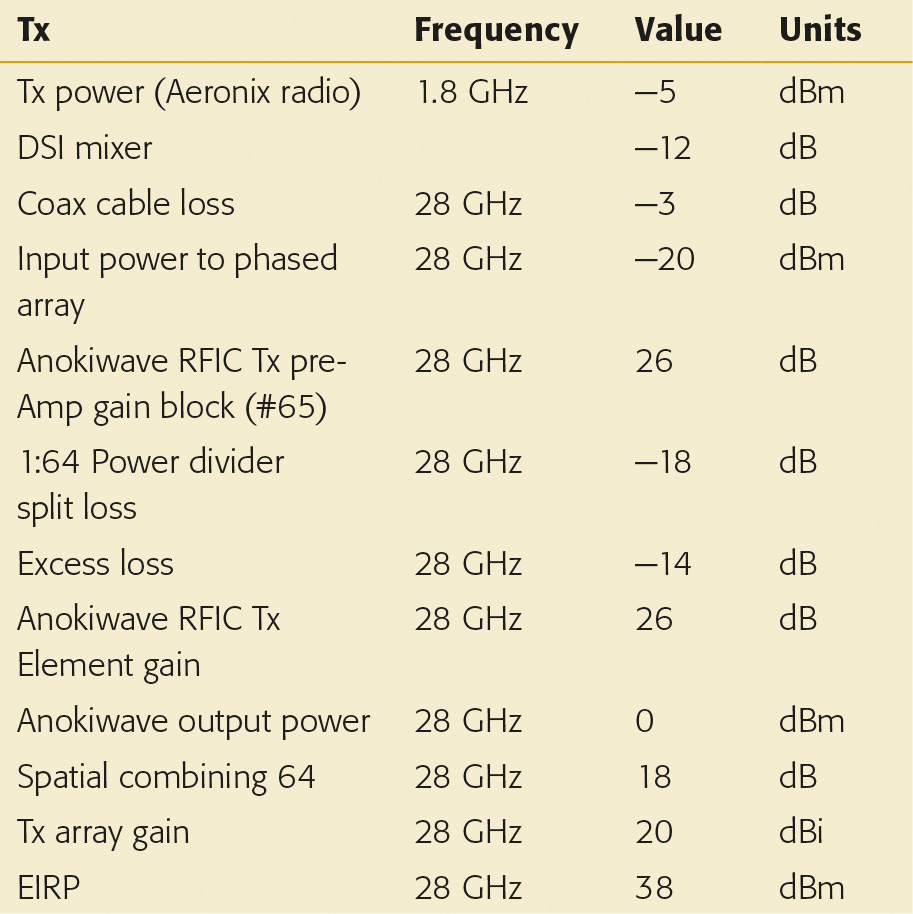
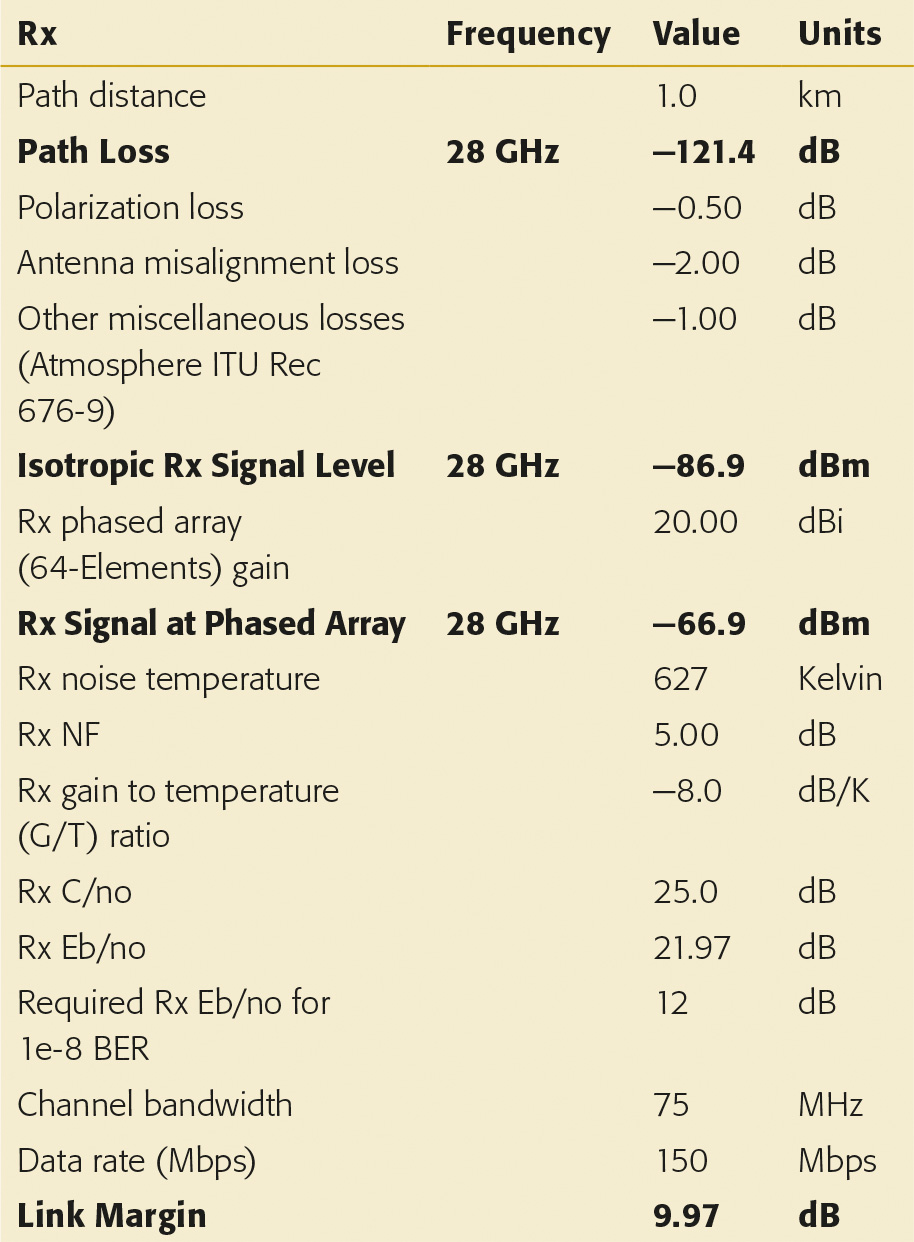
Table 2 shows the transmit link budget, with an EIRP of approximately 38 dBm, and a 4-dB back-off from the compressed EIRP. This correlates with our measured EIRP from the “Phased-Array Antenna Development” section. Table 2 includes various factors that affect the link budget, such as transmit power, mixer loss, coaxial cable loss, input power, RFIC’s Tx preamp gain block, power divider split loss, excess loss, RFIC Tx-element gain, RFIC output power, spatial combining, and Tx array gain. Similarly, Table 3 shows the Rx link budget, and includes estimated factors such as path loss, polarization misalignment loss, and atmospheric loss. The beamformer chip has an associated NF and, using that, we can also estimate the G/T, as shown in Table 3. Once that is known, we can assume the characteristics of the waveform, which in this case is a QPSK with a 75-MHz bandwidth. We can determine what carrier-to-noise ratio (C/No or CNR), or equivalently the Eb/No which is the CNR per bit, is required given a required bit-error-rate (BER). We estimate that our link margin is approximately 10 dB, which means that we could likely operate these arrays at distances approaching 10 km. To add precision to the link budget estimate, one could also measure the G/T of the phased arrays using the hot and cold method described in [12]. This would add fidelity in the link budget estimate.

Table 2. Tx link budget for QPSK.

Table 3. Rx link budget for QPSK.

Conclusion

We have presented the development and demonstration of a Ka-band T/R phased-array antenna that is capable of maintaining a 1.0 km OTA link, with up to 150-Mb/s data rate at electronically scanned angles up to 60°. We also describe test and evaluation methods for performing pertinent OTA microwave LOS digital communications leveraging these phased-array antennas.

Acknowledgment

This work was supported in part by the Army C5ISR Center. The authors would like to thank Nhat Truong of San Diego State University for his support during the test.

References

[1] S. K. Sharma and J.-C. S. Chieh, Multifunctional Antennas and Arrays for Wireless Communication Systems. Piscataway, NJ, USA: Wiley-IEEE Press, Apr. 2021.

[2] J.-C. S. Chieh et al., “Development of flat panel active phased array antennas using 5G silicon RFICs at Ku- and Ka-bands,” IEEE Access, vol. 8, pp. 192,669–192,681, Oct. 2020, doi: 10.1109/ACCESS.2020.3032841.

[3] K. Kibaroglu, M. Sayginer, and G. M. Rebeiz, “A low-cost scalable 32-element 28-GHz phased array transceiver for 5G communication links based on a 2x2 beamformer flip-chip unit cell,” IEEE J. Solid-State Circuits, vol. 53, no. 5, pp. 1260–1274, May 2018, doi: 10.1109/JSSC.2018.2791481.

[4] Y. Yin, T. Phelps, B. Ustundag, K. Kibaroglu, M. Sayginer, and G. M. Rebeiz, “A 1 Gbps 3.5-4.75 km communication link based on a 5G 28 GHz 8 × 8 phased-array,” in Proc. IEEE Int. Symp. Phased Array Syst. Technol. (PAST), Waltham, MA, USA, 2019, pp. 1–4, doi: 10.1109/PAST43306.2019.9020881.

[5] K. Kibaroglu, M. Sayginer, T. Phelps, and G. M. Rebeiz, “A 64-element 28-GHz phased-array transceiver with 52-dBm EIRP and 8–12-Gb/s 5G link at 300 meters without any calibration,” IEEE Trans. Microw. Theory Techn., vol. 66, no. 12, pp. 5796–5811, Dec. 2018, doi: 10.1109/TMTT.2018.2854174.

[6] A. Nafe, M. Sayginer, K. Kibaroglu, and G. M. Rebeiz, “2× 64-element dual-polarized dual-beam single-aperture 28-GHz phased array with 2× 30 Gb/s links for 5G polarization MIMO,” IEEE Trans. Microw. Theory Techn., vol. 68, no. 9, pp. 3872–3884, Sep. 2020, doi: 10.1109/TMTT.2020.2989117.

[7] J.-C. S. Chieh, E. Yeo, M. Kerber, and R. Olsen, “Ku-band dual polarized phased array utilizing silicon beamforming chipsets,” in Proc. IEEE Topical Workshop Internet Space (TWIOS), Orlando, FL, USA, 2019, pp. 1–3, doi: 10.1109/TWIOS.2019.8724517.

[8] S. Wang and G. M. Rebeiz, “Dual-band 28- and 39-GHz phased arrays for multistandard 5G applications,” IEEE Trans. Microw. Theory Techn., vol. 71, no. 1, pp. 339–349, Jan. 2023, doi: 10.1109/TMTT.2022.3220272.

[9] X. Liu et al., “Area-efficient 28-GHz four-element phased-array transceiver front-end achieving 25.2% Tx efficiency at 15.68-dBm output power,” IEEE Trans. Microw. Theory Techn., vol. 71, no. 2, pp. 654–668, Feb. 2023, doi: 10.1109/TMTT.2022.3207990.

[10] D. Zhao et al., “A K-band hybrid-packaged temperature-compensated phased-array receiver and integrated antenna array,” IEEE Trans. Microw. Theory Techn., vol. 71, no. 1, pp. 409–423, Jan. 2023, doi: 10.1109/TMTT.2022.3225289.

[11] P. Guan et al., “A 33.5–37.5-GHz four-element phased-array transceiver front-end with hybrid architecture phase shifters and gain controllers,” IEEE Trans. Microw. Theory Techn., early access, 2023, doi: 10.1109/TMTT.2023.3248175.

[12] A. Alhamed, G. Gültepe, and G. M. Rebeiz, “64-element 16–52-GHz transmit and receive phased arrays for multiband 5G-NR FR2 operation,” IEEE Trans. Microw. Theory Techn., vol. 71, no. 1, pp. 360–372, Jan. 2023, doi: 10.1109/TMTT.2022.3200415.

[13] O. Kazan, Z. Hu, L. Li, A. Alhamed, and G. M. Rebeiz, “An 8-channel 5–33-GHz transmit phased array beamforming IC with 10.8–14.7-dBm Psat for C-, X-, Ku-, and Ka-band SATCOM,” IEEE Trans. Microw. Theory Techn., vol. 71, no. 5, pp. 2029–2039, May 2023, doi: 10.1109/TMTT.2022.3226441.

[14] Y. Yin, Q. Ma, and G. M. Rebeiz, “A 14-17.2 GHz dual-polarized 256-element transmit phased-array with 40 dBW EIRP,” in Proc. IEEE Int. Symp. Phased Array Syst. Technol. (PAST), Waltham, MA, USA, 2022, pp. 1–4, doi: 10.1109/PAST49659.2022.9974988.

[15] K. K. W. Low, T. Kanar, S. Zihir, and G. M. Rebeiz, “A 17.7–20.2-GHz 1024-element K-band SATCOM phased-array receiver with 8.1-dB/K G/T, ±70° beam scanning, and high transmit isolation,” IEEE Trans. Microw. Theory Techn., vol. 70, no. 3, pp. 1769–1778, Mar. 2022, doi: 10.1109/TMTT.2022.3142275.

[16] S. Li, Z. Zhang, and G. M. Rebeiz, “An eight-element 136–147 GHz wafer-scale phased-array transmitter with 32 dBm peak EIRP and >16 Gbps 16QAM and 64QAM operation,” IEEE J. Solid-State Circuits, vol. 57, no. 6, pp. 1635–1648, Jun. 2022, doi: 10.1109/JSSC.2022.3148385.

[17] S. Li, Z. Zhang, B. Rupakula, and G. M. Rebeiz, “An eight-element 140-GHz wafer-scale IF beamforming phased-array receiver with 64-QAM operation in CMOS RFSOI,” IEEE J. Solid-State Circuits, vol. 57, no. 2, pp. 385–399, Feb. 2022, doi: 10.1109/JSSC.2021.3102876.

[18] D. del Rio et al., “A D-band 16-element phased-array transceiver in 55-nm BiCMOS,” IEEE Trans. Microw. Theory Techn., vol. 71, no. 2, pp. 854–869, Feb. 2023, doi: 10.1109/TMTT.2022.3203709.

[19] J.-C. S. Chieh, E. Yeo, R. Farkouh, M. Kerber, N. Stevens, and R. Olsen, “A 0.4 km 30 Mbps Ku-Band RF link based on a 64-element transmit and receive phased array antenna,” in Proc. IEEE Int. Symp. Phased Array Syst. Technol. (PAST), Waltham, MA, USA, 2022, pp. 1–7, doi: 10.1109/PAST49659.2022.9975062.

[20] Intelligent Gain Block Ku-Band Silicon IC, Anokiwave, San Diego, CA, USA, AWMF-0116, 2017.

[21] N257/n258 Silicon 5G Tx/Rx Quad Core IC, Anokiwave, San Diego, CA, USA, AWMF-0162, 2018.

[22] 24.0 GHz to 29.5 GHz Transmit/Receive Dual Polarization Beamformer, Analog Devices, Norwood, MA, USA, ADMV4828, 2021.

[23] 8-Channel Transmit Active Beamforming IC for Ka-Band SATCOM, Renesas Electron. Corp., Tokyo, Japan, 2021.

[24] 8-Channel Dual-Polarization TRX Beamformer IC at 26.5 GHz - 29.5 GHz, Renesas Electron. Corp., Tokyo, Japan, 2021.

[25] 8-Channel Beamforming Front End, 24.25–27.5 GHz, pSemi, San Diego, CA, USA, 2022.

[26] A. A. Serra, P. Nepa, G. Manara, G. Tribellini, and S. Cioci, “A wide-band dual-polarized stacked patch antenna,” IEEE Antennas Wireless Propag. Lett., vol. 6, pp. 141–143, Apr. 2007, doi: 10.1109/LAWP.2007.893101.

[27] S. D. Targonski, R. B. Waterhouse, and D. M. Pozar, “Design of wide-band aperture-stacked patch microstrip antennas,” IEEE Trans. Antennas Propag., vol. 46, no. 9, pp. 1245–1251, Sep. 1998, doi: 10.1109/8.719966.

[28] S. Gao, L. W. Li, M. S. Leong, and T. S. Yeo, “A broad-band dual-polarized microstrip patch antenna with aperture coupling,” IEEE Trans. Antennas Propag., vol. 51, no. 4, pp. 898–900, Apr. 2003, doi: 10.1109/TAP.2003.811080.

[29] R. B. Waterhouse, “Design of probe-fed stacked patches,” IEEE Trans. Antennas Propag., vol. 47, no. 12, pp. 1780–1784, Dec. 1999, doi: 10.1109/8.817653.

[30] S. Das et al., “A flat-panel 8 × 8 wideband K-/Ka-band dual circularly polarized phased array antenna for CubeSat communications,” IEEE Trans. Antennas Propag., vol. 71, no. 5, pp. 4153–4166, May 2023, doi: 10.1109/TAP.2023.3255640.

[31] R. Banerjee, S. K. Sharma, and S. Das, “Design of dual circular polarized wideband stacked patch flat panel phased array antenna using Ka-band 5G silicon RFICs in beamforming network,” in Proc. IEEE 19th Int. Symp. Antenna Technol. Appl. Electromagn. (ANTEM), Winnipeg, MB, Canada, 2021, pp. 1–2, doi: 10.1109/ANTEM51107.2021.9519083.

[32] “Finite antenna array analysis with a unite cell domain decomposition method,” Ansys, Canonsburg, PA, USA, Tech. Paper, 2013. [Online] . Available: https://www.padtinc.com/wp-content/uploads/2022/02/ANSYS-Finite-Antenna-Array-Analysis-with-a-Unit-Cell-Domain-Decomposition-Method-Application-Brief.pdf

[33] K. Woelder and J. Granholm, “Cross-polarization and sidelobe suppression in dual linear polarization antenna arrays,” IEEE Trans. Antennas Propag., vol. 45, no. 12, pp. 1727–1740, Dec. 1997, doi: 10.1109/8.650190.

[34] X. Gu et al., “Development, implementation, and characterization of a 64-element dual-polarized phased-array antenna module for 28-GHz high-speed data communications,” IEEE Trans. Microw. Theory Techn., vol. 67, no. 7, pp. 2975–2984, Jul. 2019, doi: 10.1109/TMTT.2019.2912819.

Digital Object Identifier 10.1109/MMM.2023.3294878

IEEE microwave magazine   October 2023